For the last two years, we have explored how to create hands-on models to help people learn math and science. Our book 3D Printed Science Projects was published by Apress in 2016, with a second volume in late spring 2017. Each chapter explains a scientific or mathematical principle, and then develops 3D printable models, many of which can be changed to demonstrate different cases. We found that creating these models was difficult and required both deep knowledge of the science and of the best ways to create a 3D printable model.
We now want to take this to the next level and figure out how to restructure learning a math subject completely, aiming at self-learning and at supporting learners who do much better at a subject if they can make and hold something. There are a lot of one-off projects to demonstrate a particular concept (and we have those in our books) but now we want to see how to think differently given the tools available to us.
Here is our video summarizing the project so far:
We talk to many self-taught hackers who are excellent intuitive engineers, but for whatever reason never took calculus. Calculus is a gateway to a deep understanding of physics and more advanced mathematics. There are online classes which can be taken, in some cases for free, but they are traditional algebra-heavy classes as far as we have seen. What is out there for learning advanced math and physics using 3D printing and open source electronics?
There are databases of 3D printable objects but these are not curated and the quality can be uneven. The science or math in free database models can be just plain wrong. Most commonly 3D printable math models are esoteric “math zoos” that look awesome but give no insight into how the model fits into a bigger picture. The lack of curated, rationally-organized 3D printable math and science models, and the difficulty of creating them, has held back use of 3D printing as an educational tool, even where it might be very effectively applied. Electronics projects are even more scattershot in this way.
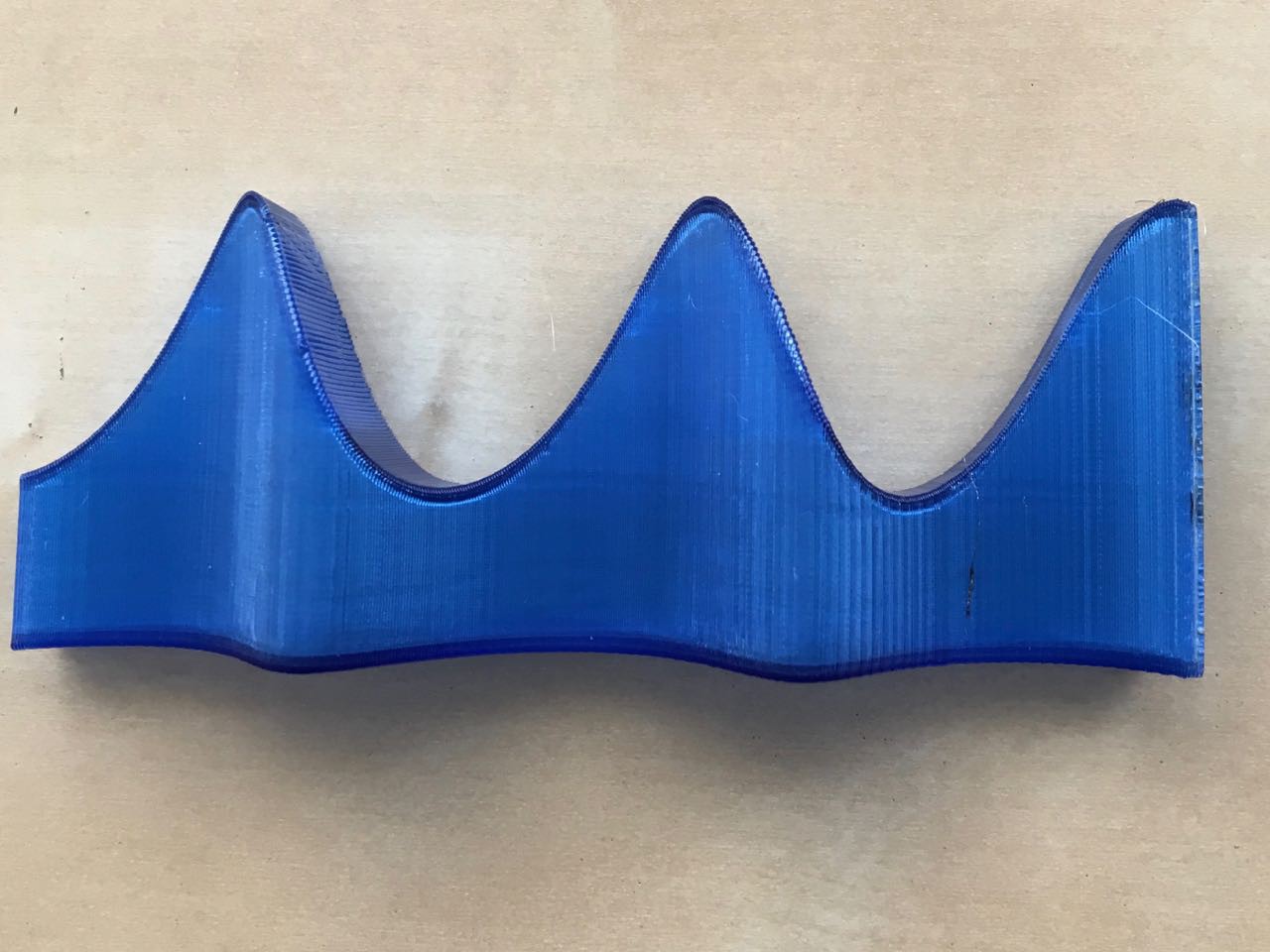
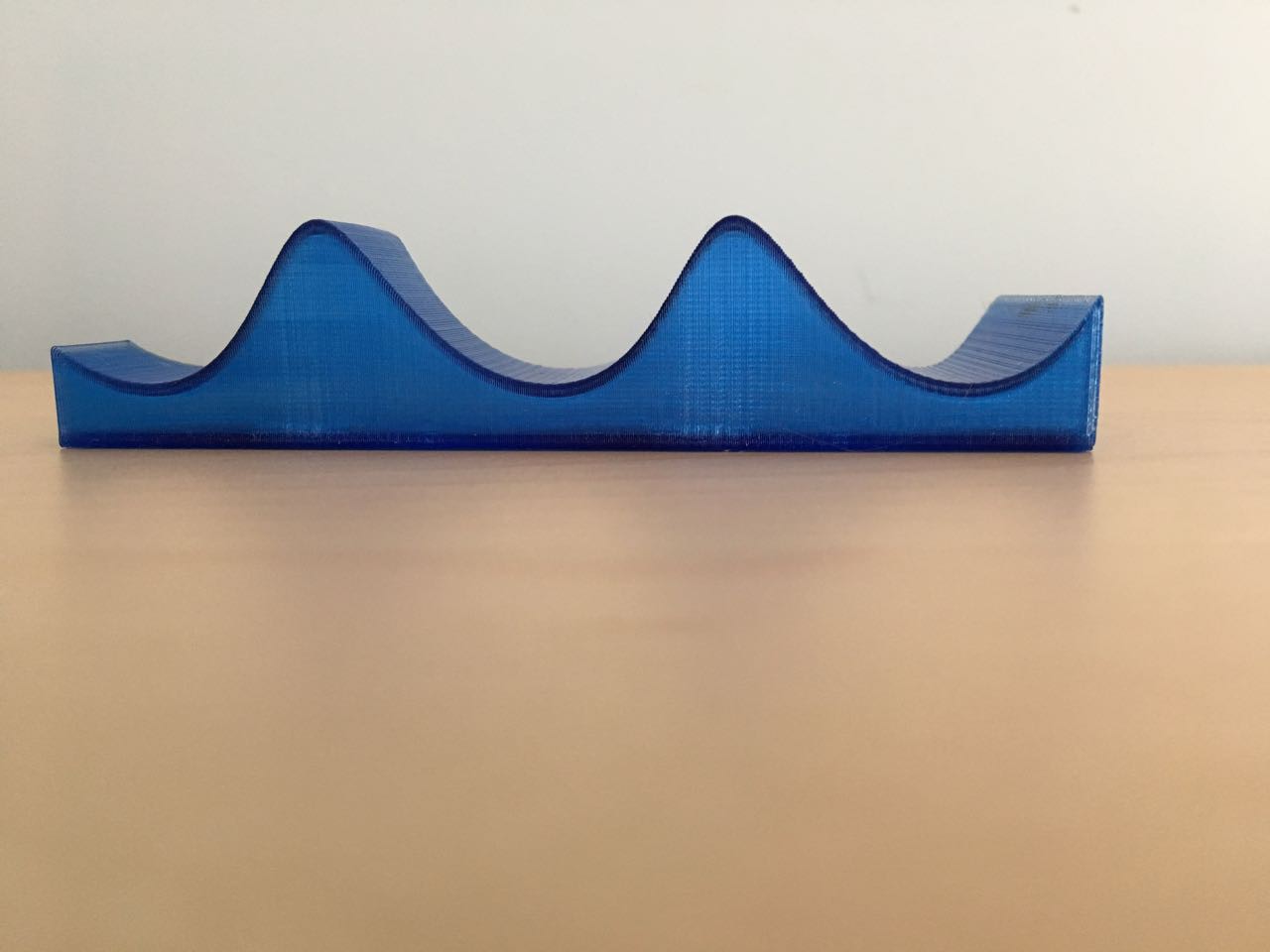
 Kepler's laws in plastic- 3D printed orbits
Kepler's laws in plastic- 3D printed orbits
As an example, our project image is “Kepler’s Laws in Plastic." The models are available for download at our publisher's page (linked above) for the 2016 3D Printed Science Projects book. They depict orbits (Earth, Venus, Mercury in one set, Halley’s Comet to a different scale in the other) with the height being the velocity of the body at that point in its orbit. Kepler developed his Laws about these relationships in about 1609, without benefit of computers, 3D printers (or calculus.) He had to rely on a purely geometrical approach. Although Joan was a JPL rocket scientist for 16 years, when we created this model both of us developed new insights. We want to bring many people those insights and make math as natural as using construction toys. We have been surprised at the emotional response this project has generated in many people (see our narrative in our logs) and we are excited about where this might go.
What Will Our Project Do?
We have gone back and looked at the oldest roots of calculus as we know it - Isaac Newton’s Philosophiae Naturalis Principia Mathematica, usually called Principia. Pages of Newton’s copy with his notes on it (with his papers at Cambridge University in England) can be seen here. Kindle versions of English translations are available starting at 99 cents.
One thing that struck us was that the entire work has only geometry. The emphasis on algebraic forms came later on. There were good reasons for this, but our idea is this:If we go back to the source, knowing what we know now, can we create a mashup across three and a half centuries that Newton himself might have appreciated? We want to find good intuitive starting points to teach calculus-as-physics and then find hackerish ways to teach them in a hands-on way.
Initially we need to define a set of core concepts that best lend themselves to this...
Read more » Joan Horvath
Joan Horvath

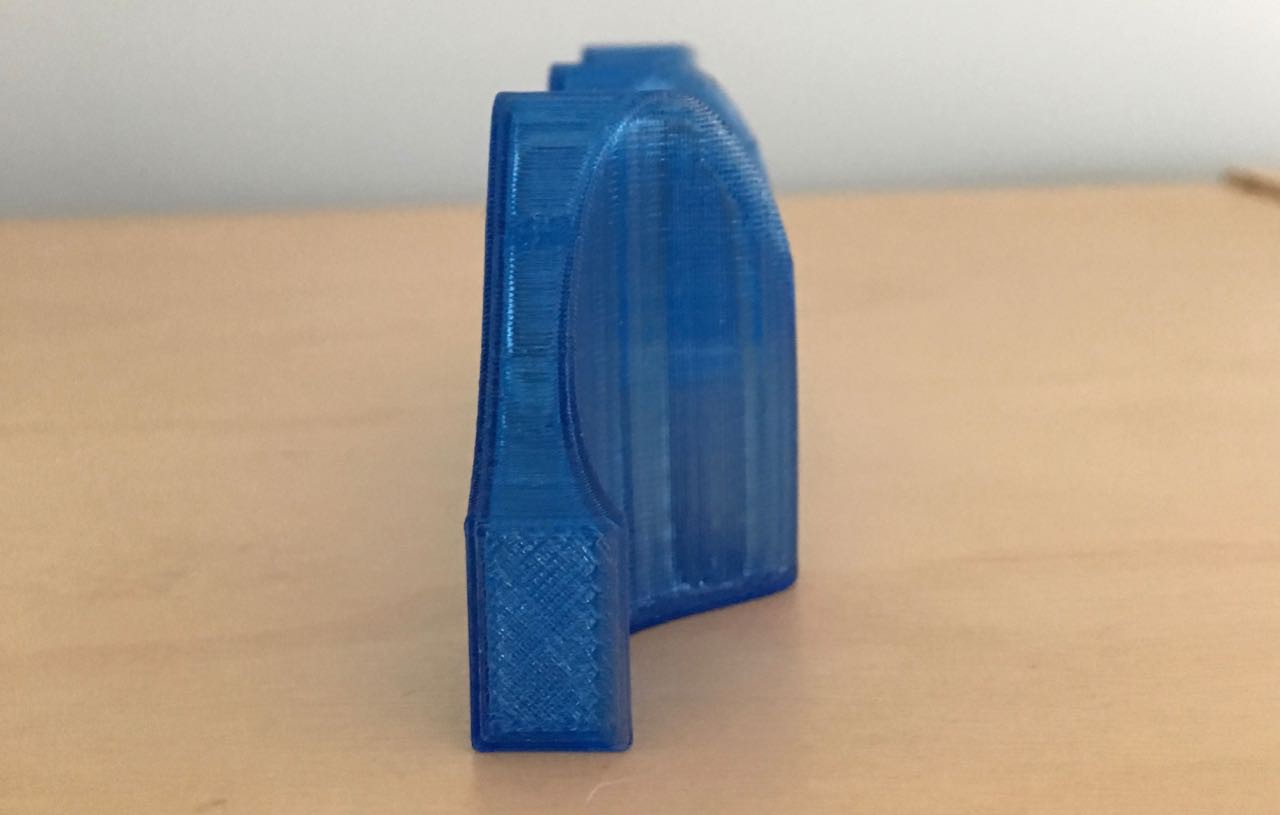
 Expanding Lotke-Volterra equations solution
Expanding Lotke-Volterra equations solution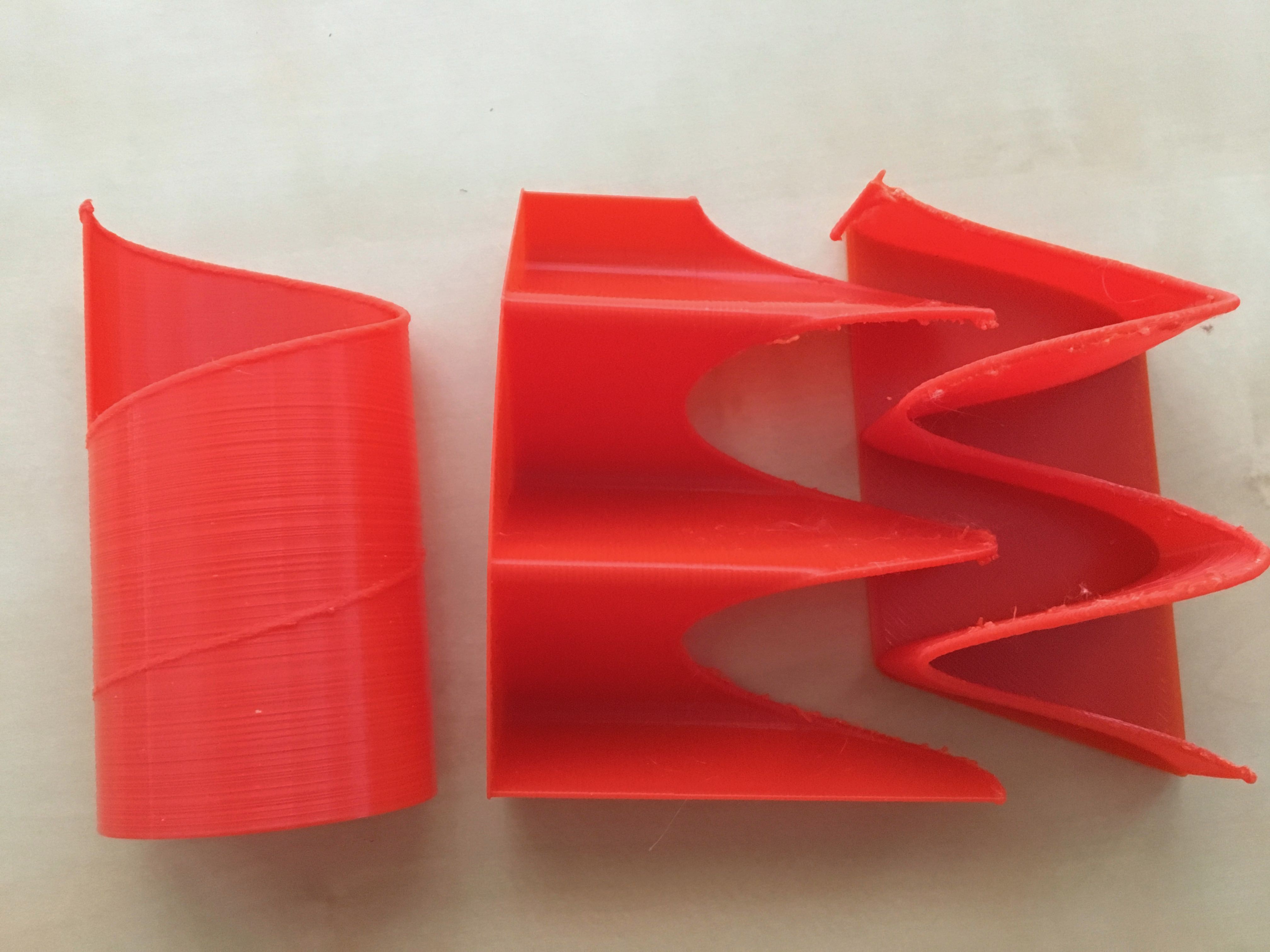 Three versions of the visualization, printed with different axis "up" during printing.
Three versions of the visualization, printed with different axis "up" during printing. 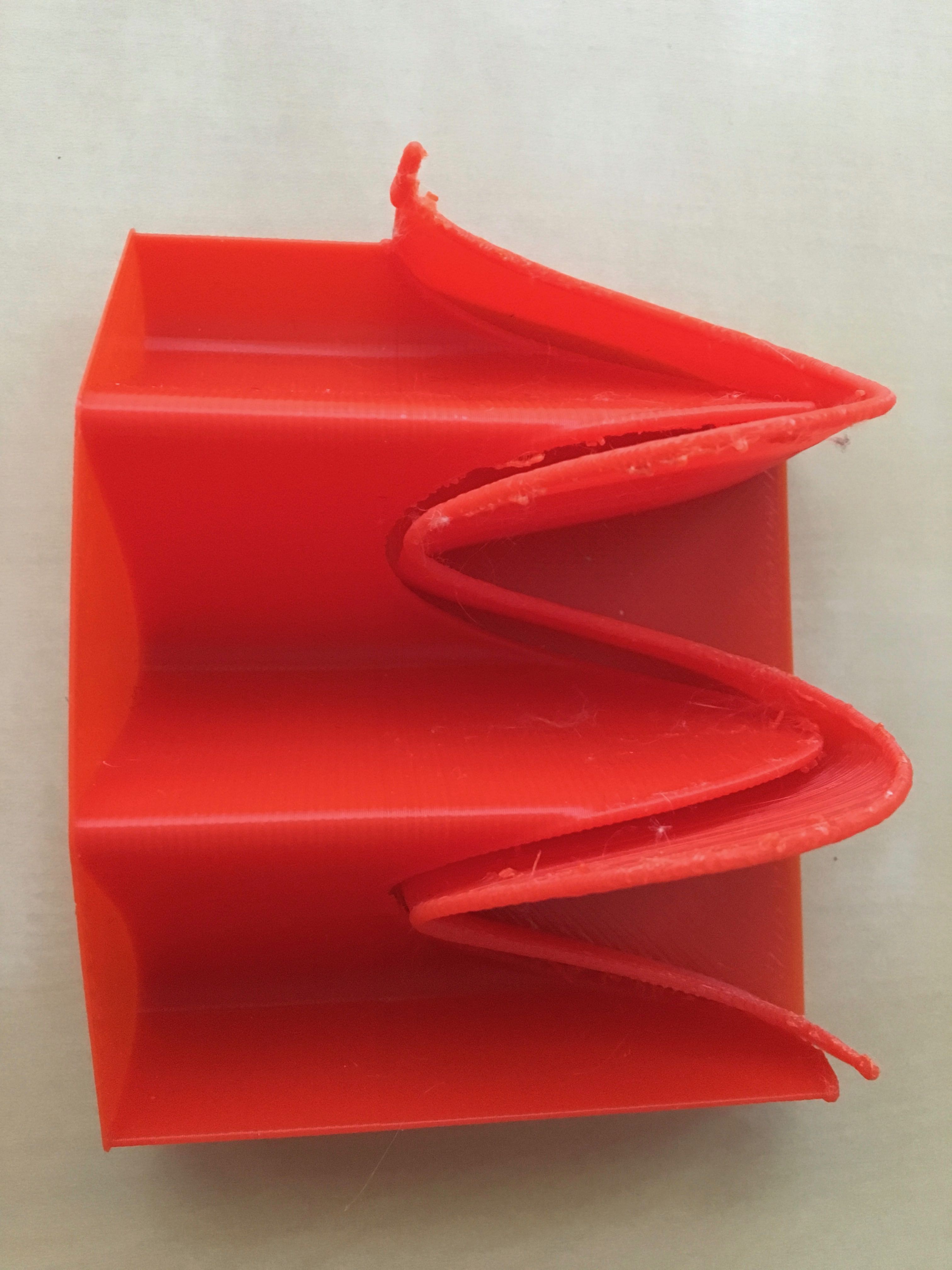

 Sinusoids and exponentials
Sinusoids and exponentials






 Jaime García
Jaime García

 Giovanni Leal
Giovanni Leal
I appreciate what you're trying to do with the physical geometry and hope that you make further progress.
As an example for you, I was one of those students that struggled linking Algebra, Trigonometry, and Calculus concepts fast enough to pass the 50-minute tests and ended up having to take pre-Calculus twice, Calculus I four times, and Calculus III twice before I was able to find a way to pass them.
I almost thew in the towel on my 4th attempt at Calculus, but one summer in the early 1990's, a retired dean of mathematics from an engineering college was upset at how it had been taught to us. Since the classes were 2+ hours in length, he had enough time to speak about things outside of the textbook, such as his joy of mathematics, and he even brought in plastic blocks and assembled them so that we could visualize the dimensional progression, including the 4th dimension, and explained how this progression to even higher dimensions was the same. He even discussed mysterious concepts like the possible quantization of time. This gave me the motivation I needed to go on.
I gave up four classes later, though, when I hit Differential Equations, due to the abstract, complex language and patterns, but have recently taken a modern class in complexity science with a qualitative approach that skips the complex differentiation, so that I could see patterns that were never shown to me.
I absolutely agree that making these patterns accessible at the very start is extremely important in inspiring people like me to proceed. If you're goal-oriented, you are often motivated to learn difficult concepts needed to reach that goal if you are certain the goal is worthwhile, but placing an algebraic minefield in front of us without showing us what is on the other side of that hill will have obvious results.
Here are some of the difficulties that have to be surmounted to learn Calculus as an English speaker:
First one has to learn "mathematical English", the meanings for the same words differing between common English, philosophical English, and mathematics. This can be even more confusing (which it was in some of my cases) if your instructor is not strong in common English.
Then one has to learn algebraic expressions, which are very compressed and have shape-shifting forms, assigning different meanings to the same symbols, depending on context, which gets highly abstract, and then you have to "decompress" it into mathematical English for class discussion.
And then this problem is worsened by the various notations for differentiation:
https://en.wikipedia.org/wiki/Notation_for_differentiation
As I would repeat the coursework, my different books/professors switched between Leibniz, Lagrange and its variations without consideration of what I had used before. Most of the mental difficulties are in overcoming layers of memorizing/decompressing trivial abstractions that are not related to the mathematical patterns under study.
It would be wonderful if you could find a way to simplify the algebraic issues, as this was the primary issue for me and is a requirement for any testing/advancement.
I tutored someone in College Algebra at an accredited college in recent years and was shocked to find that the teaching style where I live in the US had not improved in 30 years. In fact, it was worse. Not only did the student have to surmount the language hurdles I just mentioned, they had to feed online learning systems with correct keyboard input over unreliable Internet connections, wasting time just trying to figure out how to enter mathematical notation correctly through an idiosyncratic Javascript interpreter. And the scoring system wasn't intelligent enough to give partial credit or evaluate the method the student used to derive the answer.